Mathematics SSE

Key Unit competence: Analyse and interpret statistical 

data from daily life situations

6.0. Introductory Activity

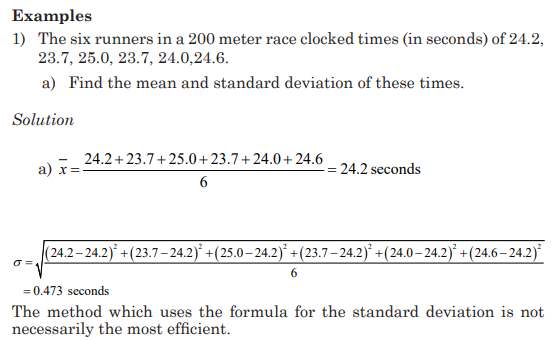
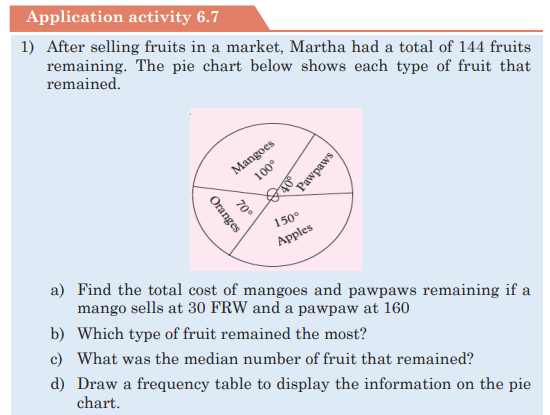
1) 1. At the market a fruit-seller has the following daily sales 

Rwandan francs for five consecutive days: 1000Frw, 1200Frw, 

125Frw, 1000Frw, and 1300Frw. Help her to determine the money 

she could get if the sales are equally distributed per day to get the 

same total amount of money

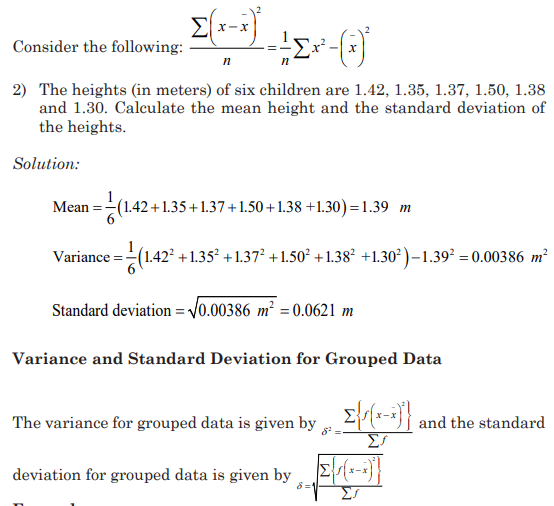
2) During the welcome test of Mathematics for the first term 10 

student-teachers of year one language education scored the 

following marks out of 10 : 3, 5,6,3,8,7,8,4,8 and 6. 

a) What is the mean mark of the class? 

b) Chose the mark that was obtained by many students.

c) Compare the differences between the mean of the group and 

the mark for every student teacher.

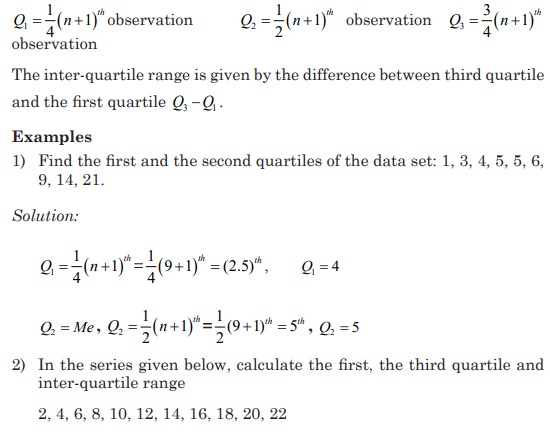
6.1 Definition and type of data

Activity 6.1

Carry out research on statistics to determine the meanings of 

statistics and types of data. Use your findings to select qualitative and 

quantitative data from this list: Male, female, tall, age, 20 sticks, 45 

student-teachers, and 20 meters, 4 pieces of chalk.

CONTENT SUMMARY

Statistics is the branch of mathematics that deals with data collection, 

data organization, summarization, analysis and draws conclusions from 

data.

The use of graphs, charts, and tables and the calculation of various 

statistical measures to organize and summarize information is 

called descriptive statistics. Descriptive statistics helps to reduce our 

information to a manageable size and put it into focus. 

Every day, we come across a wide variety of information in form of 

facts, numerical figures or table groups. A variable is a characteristic 

or attribute that can assume different values. Data are the values 

(measurements or observations) that the variables can assume.

Variables whose values are determined by chance are called random 

variables. A collection of data values forms a data set. Each value in 

the data set is called a data value or a datum. 

For example information related to profit/ loss of the school, attendance 

of students and tutors, used materials, school expenditure in term or 

year, etc. These facts or figure which is numerical or otherwise, collected 

with a definite purpose is called data. This is the word derived from Latin 

word Datum which means pieces of information. 

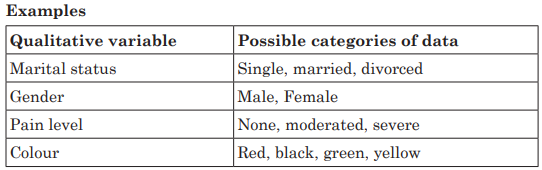
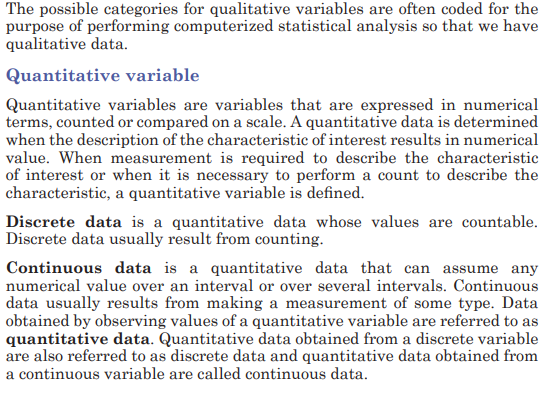
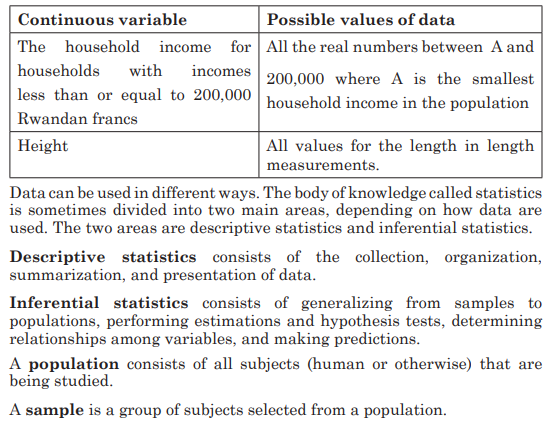
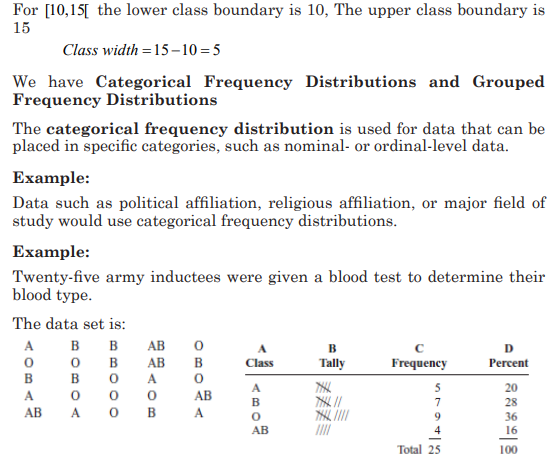
Qualitative variable

The qualitative variables are variables that cannot be expressed using 

a number. A qualitative data is determined when the description of the 

characteristic of interest results is a non-numerical value. A qualitative 

variable may be classified into two or more categories. Data obtained by 

observing values of a qualitative variable are referred to as qualitative 

data.

The three most commonly used graphs in research are

1) The histogram.

2) The frequency polygon.

3) The cumulative frequency graph or ogive (pronounced o-jive).

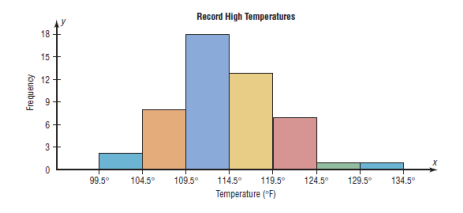
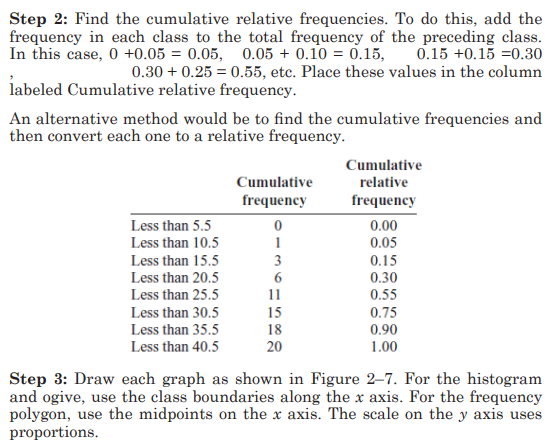
a)The Histogram

The histogram is a graph that displays the data by using contiguous 

vertical bars (unless the frequency of a class is 0) of various heights to 

represent the frequencies of the classes.

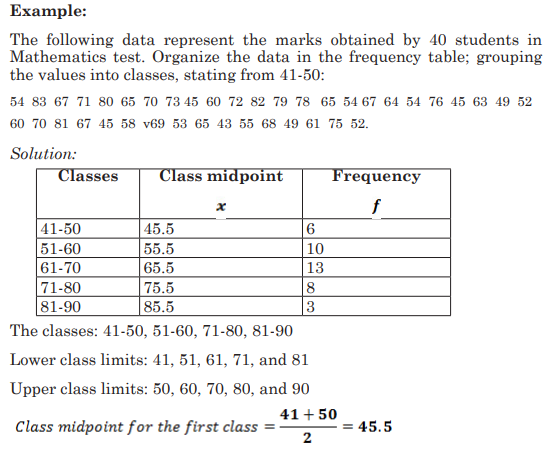
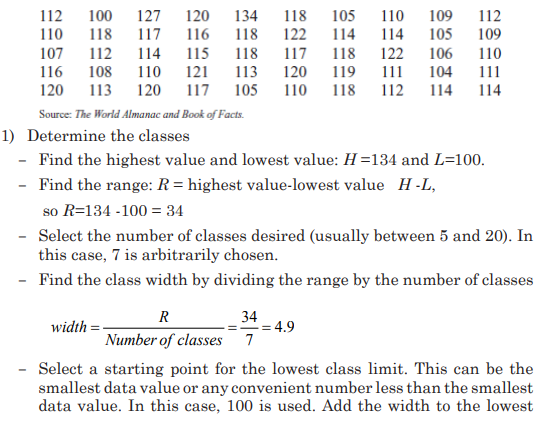
Example:

Construct a histogram to represent the data shown for the record high 

temperatures for each of the 50 states

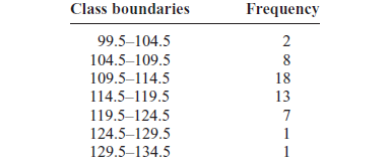
Step 1: Draw and label the x and y axes. The x axis is always the 

horizontal axis, and the y axis is always the vertical axis. 

Step 2: Represent the frequency on the y axis and the class boundaries 

on the x axis.

Step 3: Using the frequencies as the heights, draw vertical bars for each 

class. See Figure below

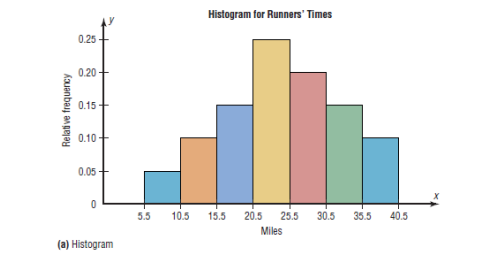
b)The Frequency Polygon

The frequency polygon is a graph that displays the data by using lines 

that connect points plotted for the frequencies at the midpoints of the 

classes. The frequencies are represented by the heights of the points. 

Example: 

Using the frequency distribution given in Example 2–4, construct a 

frequency polygon

Step 1 Find the midpoints of each class. Recall that midpoints are found 

by adding the upper and lower boundaries and dividing by 2:

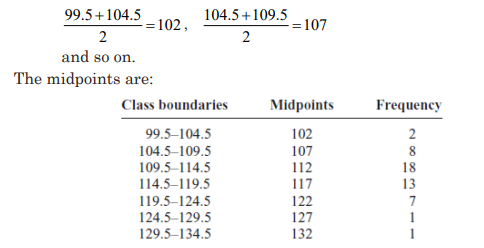
Step 2 Draw the x and y axes. Label the x axis with the midpoint of each 

class, and then use a suitable scale on the y axis for the frequencies.

Step 3 Using the midpoints for the x values and the frequencies as the y 

values, plot the points.

Step 4 Connect adjacent points with line segments. Draw a line back to 

the x axis at the beginning and end of the graph, at the same distance 

that the previous and next midpoints would be located, as shown in 

Figure 2–3

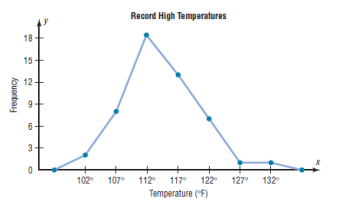
The frequency polygon and the histogram are two different ways to 

represent the same data set. The choice of which one to use is left to the 

discretion of the researcher.

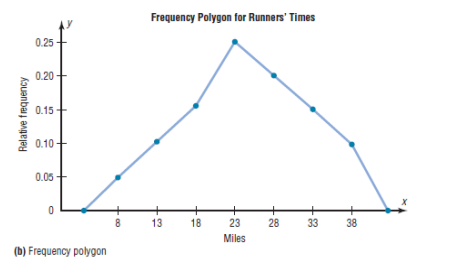
c) The Ogive

The ogive is a graph that represents the cumulative frequencies for the 

classes in a frequency distribution.

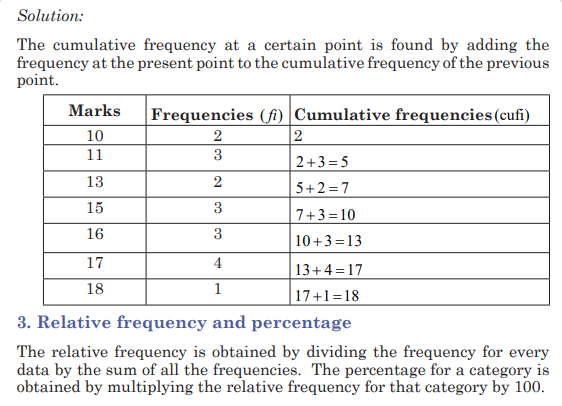
Step 1: Find the cumulative frequency for each class

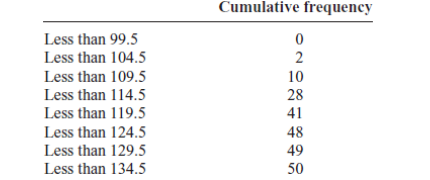
Step 2: Draw the x and y axes. Label the x axis with the class boundaries. 

Use an appropriate scale for the y axis to represent the cumulative 

frequencies.

(Depending on the numbers in the cumulative frequency columns, scales 

such as 0, 1, 2, 3, . . . , or 5, 10, 15, 20, . . . , or 1000, 2000, 3000, . . . can 

be used.

Do not label the y axis with the numbers in the cumulative frequency 

column.) In this example, a scale of 0, 5, 10, 15, . . . will be used.

Step 3 Plot the cumulative frequency at each upper class boundary, as 

shown in Figure below. Upper boundaries are used since the cumulative 

frequencies represent the number of data values accumulated up to the 

upper boundary of each class.

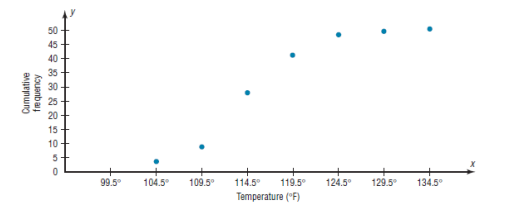
Step 4 Starting with the first upper class boundary, 104.5, connect 

adjacent points with line segments, as shown in the figure. Then extend 

the graph to the first lower class boundary, 99.5, on the x axis.

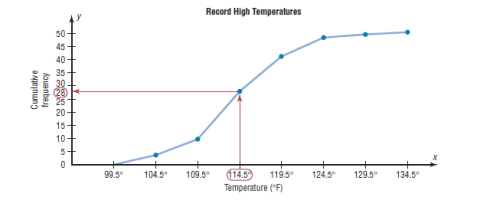
Cumulative frequency graphs are used to visually represent how many 

values are below a certain upper class boundary. For example, to find 

out how many record high temperatures are less than 114.5_F, locate 

114.5_F on the x axis, draw a vertical line up until it intersects the graph, 

and then draw a horizontal line at that point to the y axis. The y axis 

value is 28, as shown in the figure.

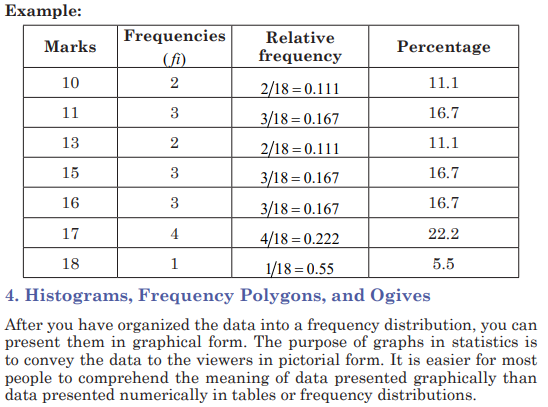
5. Relative Frequency Graphs

The histogram, the frequency polygon, and the ogive shown previously 

were constructed by using frequencies in terms of the raw data. These 

distributions can be converted to distributions using proportions instead 

of raw data as frequencies. These types of graphs are called relative 

frequency graphs.

Example:

Construct a histogram, frequency polygon, and ogive using relative 

frequencies for the distribution (shown here) of the kilometers that 20 

randomly selected runners ran during a given week.

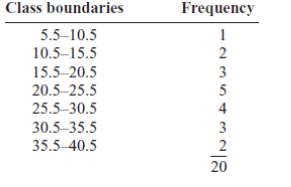
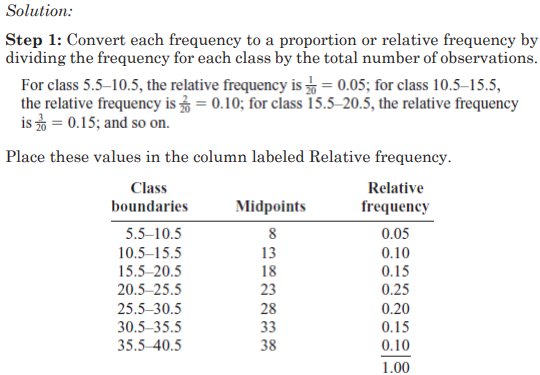
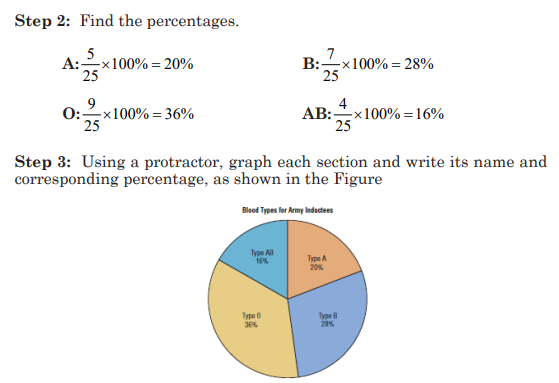
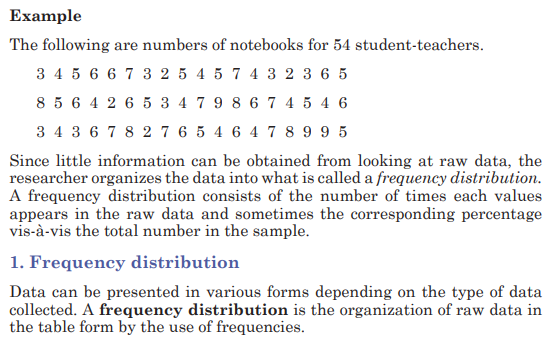
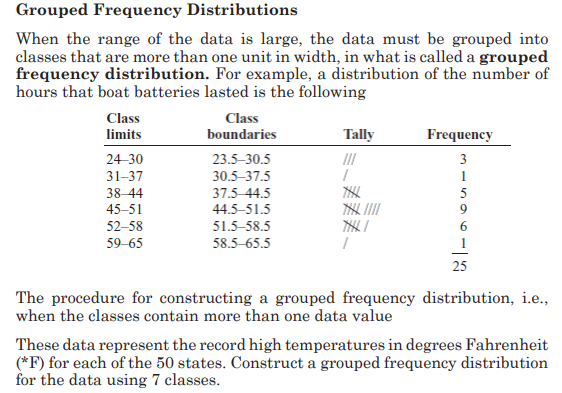
7. Stem and Leaf Plots

The stem and leaf plot is a method of organizing data and is a combination 

of sorting and graphing. It uses part of the data value as the stem and 

part of the data value as the leaf to form groups or classes. It has the 

advantage over a grouped frequency distribution of retaining the actual 

data while showing them in graphical form.

Examples: 

1) At an outpatient testing center, the number of cardiograms performed 

each day for 20 days is shown. Construct a stem and leaf plot for the 

data.

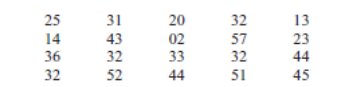
Step 1: Arrange the data in order: 02, 13, 14, 20, 23, 25, 31, 32, 32, 

32, 32, 33, 36, 43, 44, 44, 45, 51, 52, 57

Note: Arranging the data in order is not essential and can be 

cumbersome when the data set is large; however, it is helpful in 

constructing a stem and leaf plot. The leaves in the final stem and 

leaf plot should be arranged in order.

Step 2 Separate the data according to the first digit, as shown. 

02 13, 14 20, 23, 25 31, 32, 32, 32, 32, 33, 36, 43, 44, 44, 45 51, 52, 57

Step 3: A display can be made by using the leading digit as the stem 

and the trailing digit as the leaf. 

For example, for the value 32, the leading digit, 3, is the stem and 

the trailing digit, 2, is the leaf. For the value 14, the 1 is the stem and 

the 4 is the leaf. Now a plot can be constructed as follows: 

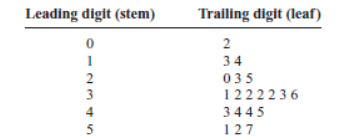
It shows that the distribution peaks in the center and that there are 

no gaps in the data. For 7 of the 20 days, the number of patients 

receiving cardiograms was between 31 and 36. The plot also shows 

that the testing center treated from a minimum of 2 patients to a 

maximum of 57 patients in any one day.

If there are no data values in a class, you should write the stem 

number and leave the leaf row blank. Do not put a zero in the leaf 

row.

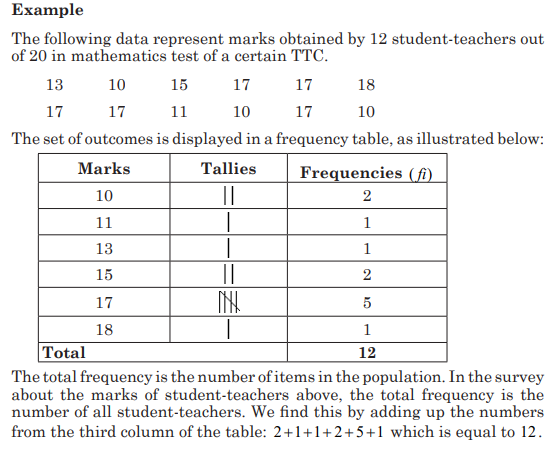
2) The mathematical competence scores of 10 student-teachers 

participating in mathematics competition are as follows: 15, 16, 21, 

23, 23, 26, 26, 30, 32, 41. Construct a stem and leaf display for these 

data by using 2, 3, and 4 as your stems.

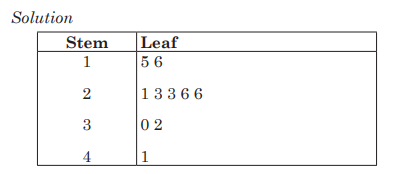
This means that data are concentrated in twenties.

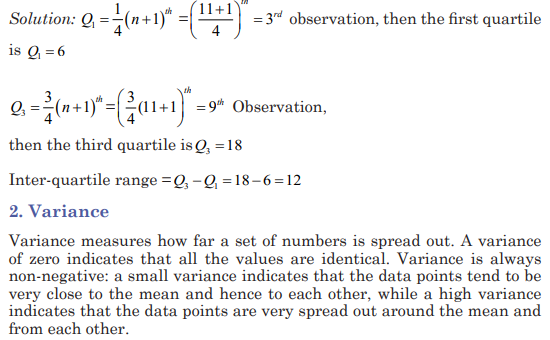
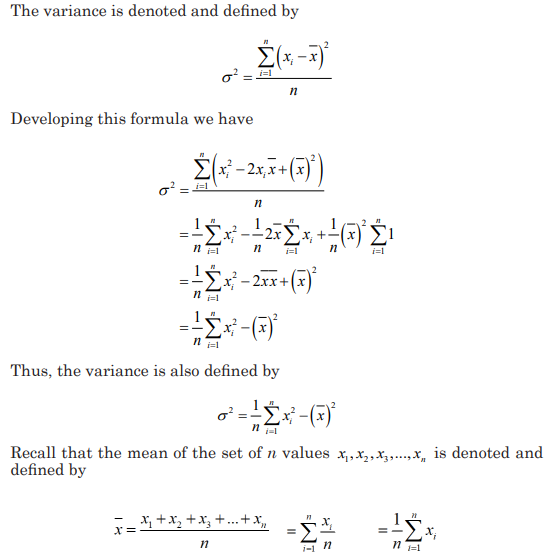
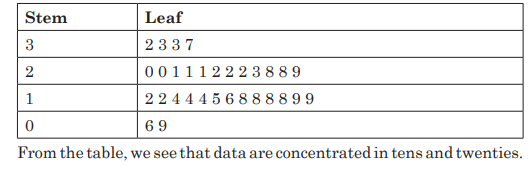
3) The following are results obtained by student-teachers in French out 

of 50. 

37, 33, 33, 32, 29, 28, 28, 23, 22, 22, 22, 21, 21, 21, 20, 

20, 19, 19, 18, 18, 18, 18, 16, 15, 14, 14, 14, 12, 12, 9, 6

Use stem and leaf to display data

Solution: 

Numbers 3, 2, 1, and 0, arranged as a stems to the left of the bars. 

The other numbers come in the leaf part.

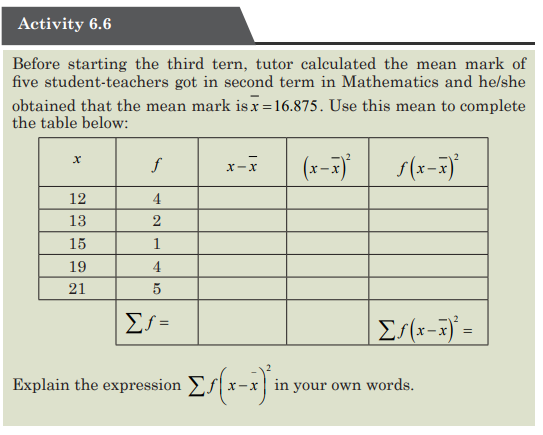
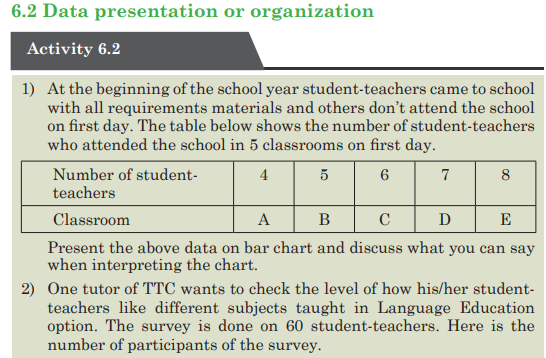
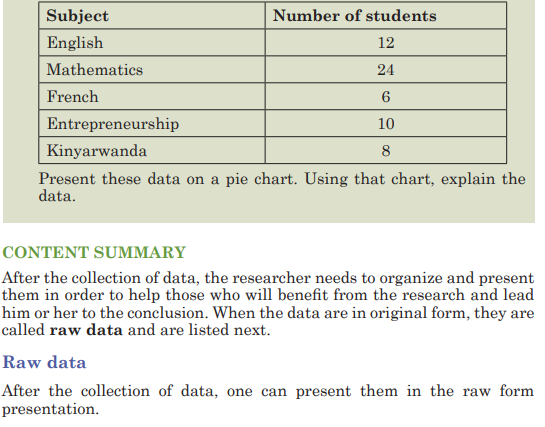
Application activity 6.2

1) Suppose that a tutor conducted a test for student-teachers and 

the marks out of 10 were as follows: 3 3 3 5 6 4 6 7 8 3 8 8 8 

10 9 10 9 10 8 10 6

a) Draw a frequency table;

b) Draw a relative frequency table and calculate percentage for 

each;

c) Present data in cumulative frequency table, hence show the 

number of student-teachers who did the test.

2) During the examination of English student-teacher got the 

following results out of 80: 54, 42, 61, 47, 24, 43, 55, 62, 30, 27, 28, 

43, 54, 46, 25, 32, 49, 73, 50, 45. 

 Present the results using stem and leaf.

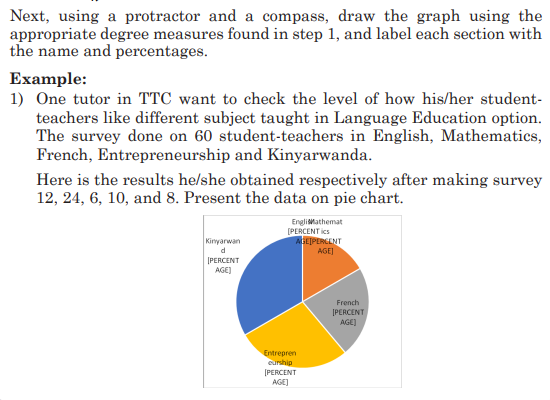
3) A firm making artificial sand sold its products in four cities: 5% 

was sold in Huye, 15% in Musanze, 15% in Kayonza and 65% was 

sold in Rwamagana.

a) What would be the angles on pie chart?

b) Draw a pie chart to represent this information. 

c) Use the pie chart to comment on these findings

6.3 Graph interpretation and Interpretation of statistical data

Activity 6.3

The graph below shows the sizes of sweaters worn by 30 year 1 

students in a certain school. Observe it and interpret it by answering 

the questions below it

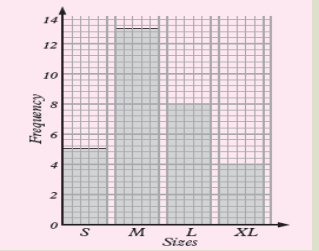
a) How many students are with small size?

b) How many students with medium size, large size and extra 

large size are there? 

CONTENT SUMMARY

Once data has been collected, they may be presented or displayed in 

various ways including graphs. Such displays make it easier to interpret 

and compare the data. 

Examples 

1) The bar graph shows the number of athletes who represented five 

African countries in an international championship.

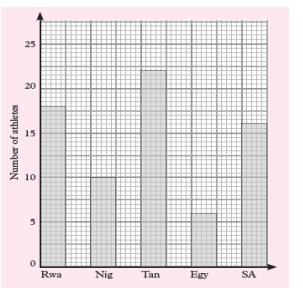
a) What was the total number of athletes representing the five 

countries? 

b) What was the smallest number of athletes representing one 

country? 

c) What was the most number of athletes representing a country? 

d) Represent the information on the graph on a frequency table.

Solution: 

We read the data on the graph: 

a) Total number of athletes are: 18 + 10 + 22 + 6 + 16 = 72 athletes 

b) 6 athletes 

c) 22 athletes

d) Representation of the given information on the graph on a 

frequency table.

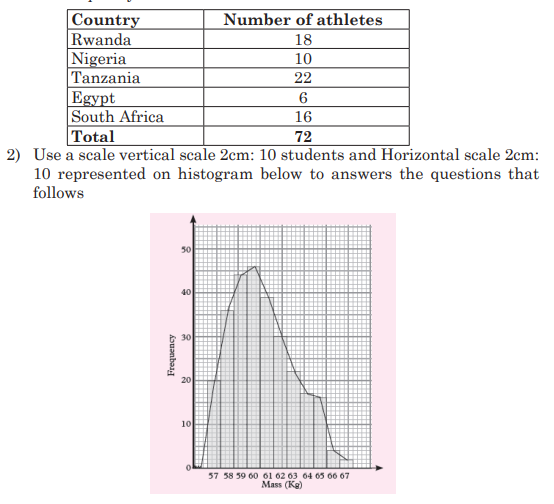
a) Estimate the mode

b) Calculate the range

Solution: 

a) To estimate the mode graphically, we identify the bar that 

represents the highest frequency. The mass with the highest 

frequency is 60 kg. It represents the mode.

b) The highest mass = 67 kg and the lowest mass = 57 kg 

Then, The range=highest mass-lowest mass=67 57 10 kg kg kg − =

Application activity 6.3

The line graph below shows bags of cement produced by CIMERWA 

industry cement factory in a minute.

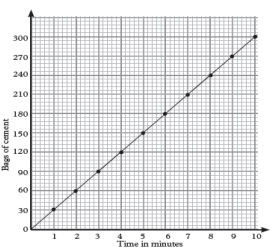
a) Find how many bags of cement will be produced in: 8 minutes, 

3 minutes12 seconds, 5 minutes and 7 minutes. 

b) Calculate how long it will take to produce: 78 bags of cement.

c) Draw a frequency table to show the number of bags produced 

and the time taken.

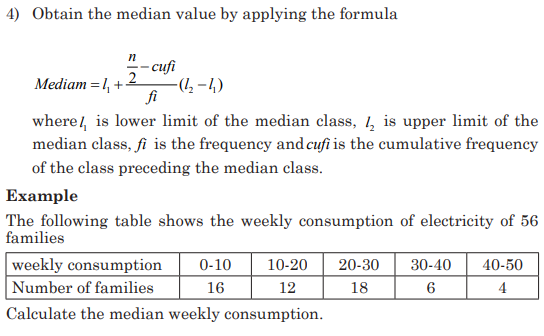
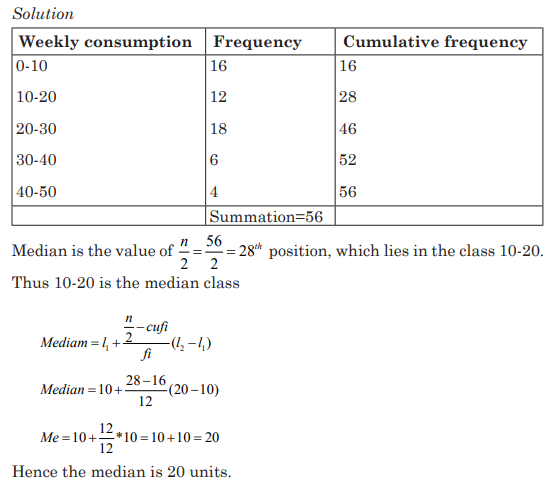
6.4 Measures of central tendencies for ungrouped data

Activity 6.4

Conduct research in the library or on the internet and explain 

measures of central tendency, their types and provide examples. 

Insist on explaining how to determine the Mean, Mode, Median and them 

role when interpreting statistic data.

CONTENT SUMMARY

Measures of central tendency were studies in S1 and S2. 

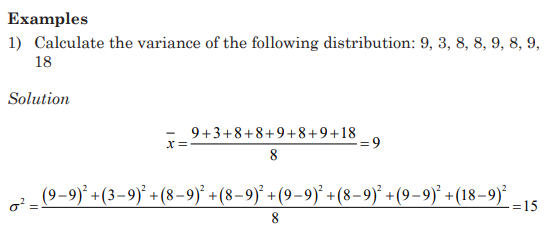
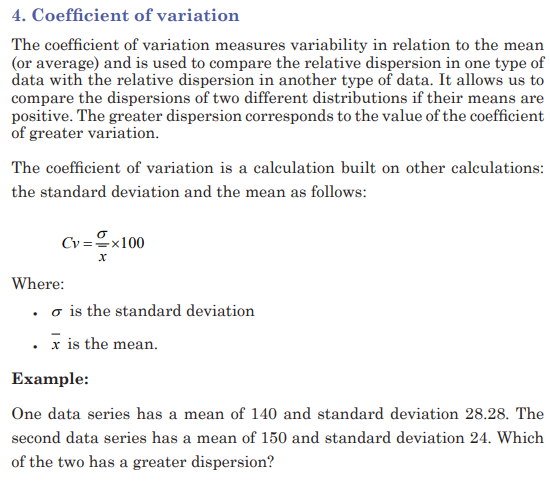
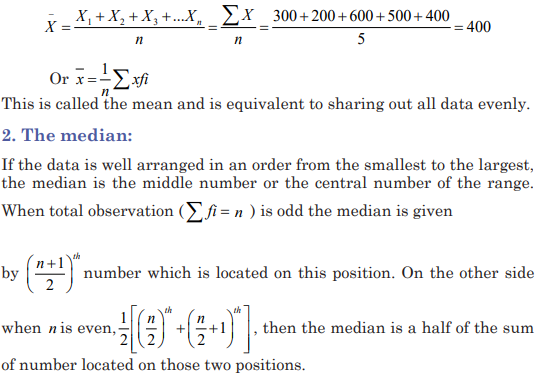
1. The mean

The mean, also known as the arithmetic average, is found by adding the 

values of the data and dividing by the total number of values.

Suppose that a fruit seller earned the flowing money from Monday to 

Friday respectively: 300, 200, 600, 500, and 400 Rwandan francs. The 

mean of this money explains the same daily amount of money that she 

should earn to totalize the same amount in 5 days.

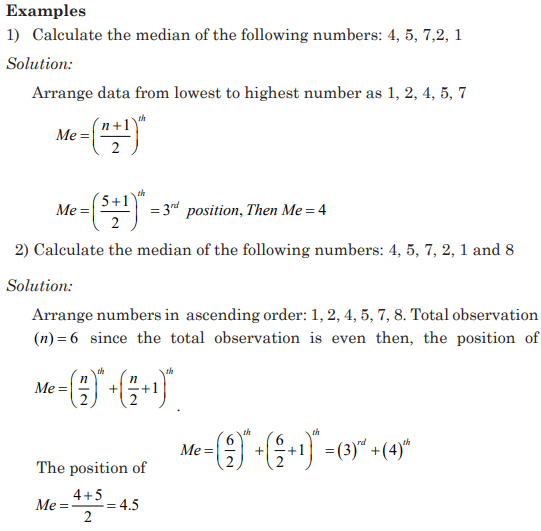
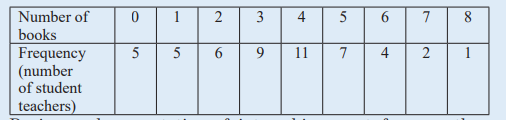
Application activity 6.4

1) A group of student-teachers from language education were asked 

how many books they had read in previous year, the results are 

shown in the frequency table below. Calculate the mean, median 

and mode of the number books read.

2) During oral presentation of internship report for year three 

student-teachers the first 10 student-teachers scored the following 

marks out of 10:

8, 7, 9, 10, 8, 9, 8, 6, 7 and 10

Calculate the mean and the median of the group.

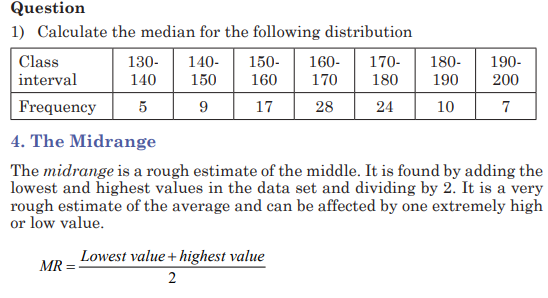
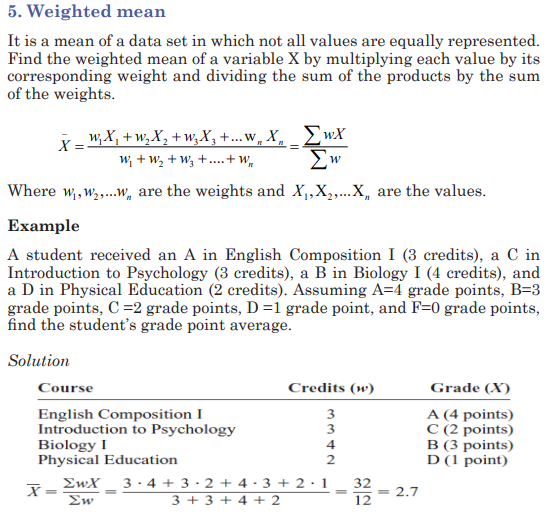
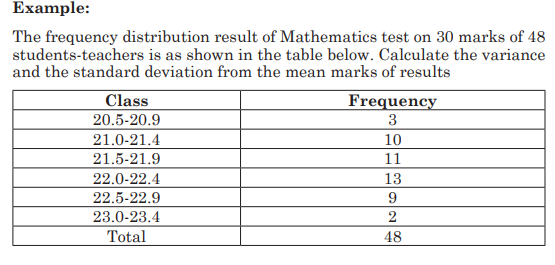
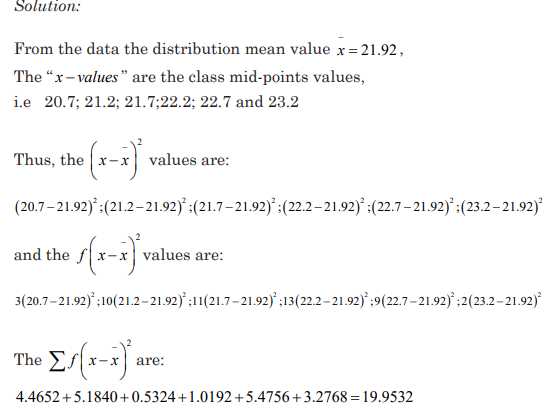
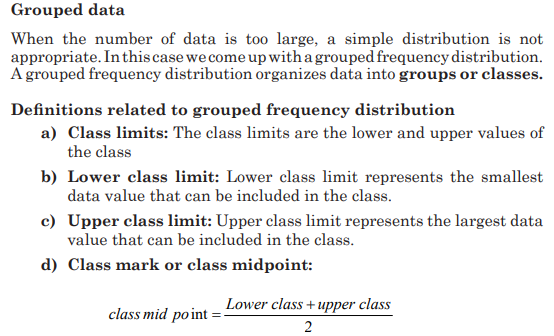
6.5 Measures of central tendencies for grouped data: 

mode, mean, median and midrange

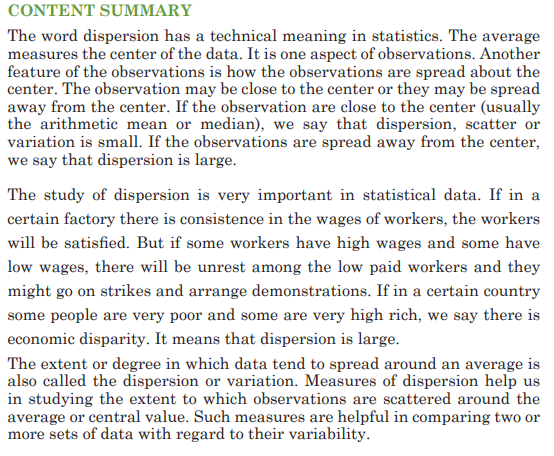
Activity 6.5

1) Conduct a research in the library or on the internet and explain 

measures of central tendency for grouped data and provide 

examples. 

Insist on explaining how to determine the Mean, Mode, Median 

and their role when interpreting statistic data. 

2) Using the frequency distribution given below, find the mean. The 

data represent the number of kilometers run during one week for 

a sample of 20 runners.

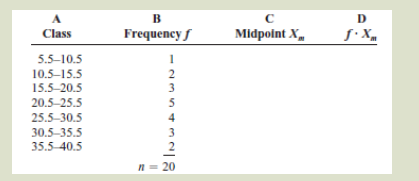
What does this mean represent considering the class in which it is 

located in the data? 

1. The mean

The process of finding the mean is the same as the one applied in the 

ungrouped data with the exception that the midpoints mx of each class in 

grouped data plays the role of x used in ungrouped data

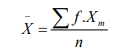
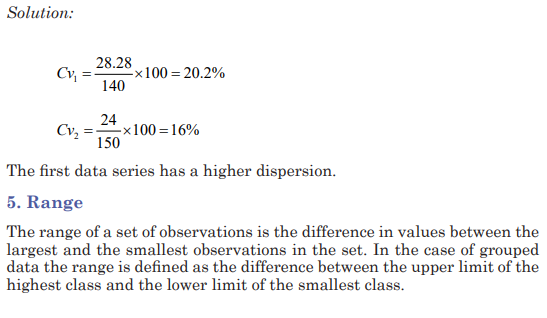
2. The mode

The mode for grouped data is the modal class. The modal class is the 

class with the largest frequency. The mode can be determined using the 

following formula:

Where: 

L: the lower limit of the modal class 

fm: the modal frequency 

f1: the frequency of the immediate class below the modal class 

f2: the frequency of the immediate class above the modal class 

w: modal class width. 

Example:

Find the modal class for the frequency distribution of kilometers that 20 

runners ran in one week.