UNIT 2:WAVE AND PARTICLE NATURE OF LIGHT
Observe the pictures A and B, and answer with scientific explanations the
following questions:
1) a) Who will absorb more heat/radiations?
b) In the dried clothes, which cloth will dry faster?
c) Basing on observations made, explain why in most schools white
shirts and blouses are preferred instead of other colours.
2) a) In packaging silvered foils are used to wrap most of fished products.
Explain why these foils are preferred instead of darkened foils.
b) Explain why it’s not recommended to paint inside one’s room with a
black paint?
c) Explain the variations in temperatures inside a house that is roofed
using black coloured iron sheets and one roofed using white ironsheets.
2.1.1. Concept of light
Particle theory of light
The nature and properties of light have been a subject of great interest and
speculation since ancient times. Until the time of Isaac Newton (1642–1727),
the Greeks believed that light consisted of tiny particles that either were
emitted by a light source or emanated from the eyes of the viewer.
Newton the chief architect of the particle theory of light held that light consisted
of tiny particles that were emitted from a light source and that these particles
stimulated the sense of sight upon entering the eye. By particle theory, he was
able to explain reflection and refraction of light.
However , derivation of the law of refraction depend on the assumption that
light travels faster in water and in glass than in air, an assumption later shown
to be false. Most scientists accepted Newton’s particle theory.
Wave theory of light
In the mid-seventeenth century, the Jesuit priest Francesco Grimaldi (1618–
1663) had observed that when sunlight entered a darkened room through a
tiny hole in a screen, the spot on the opposite wall was larger than would be
expected from geometric rays. He also observed that the border of the image
was not clear but was surrounded by colored fringes. Grimaldi attributed this
to the diffraction of light.
In 1678, one of Newton’s contemporaries, the Dutch physicist and astronomer
Christian Huygens (1629–1695), was able to explain many other properties oflight by proposing that light is a wave.
By wave theory of light, Huygens was able to explain reflection and refraction
of light by assuming that light travels more slowly in water and in glass than in
air. Huygens’ Principle is particularly useful for analyzing what happens when
waves run into an obstacle.
The bending of waves behind obstacles into the “shadow region” is known as
diffraction. Since diffraction occurs for waves, but not for particles, it can serve
as one means for distinguishing the nature of light.
In 1801, the Englishman Thomas Young (1773–1829) provided the first clear
demonstration of the wave nature of light and showed that light beams can
interfere with one another, giving strong support to the wave theory. Young
showed that, under appropriate conditions, light rays interfere with each other.
Such behaviour could not be explained at that time by a particle theory because
there was no conceivable way in which two or more particles could come
together and cancel one another.
The general acceptance of wave theory was due to the French physicist
AugustinFresnell (1788-1827), who performed extensive experiments on
interference and diffraction and put the wave theory on a mathematical basis.
In 1850, Jean Foucault measured the speed of light in water and showed thatit is less than in air, thus ruling out Newton’s particle theory.


be explained by wave theory and not by particle nature of light.
• Energy distribution in perfect black body radiation, photoelectric effect
and Compton Effect can be explained by particle nature of light and not
by wave theory. The concept of quantum mechanics is applied even to
the motion of electrons in an atom in Bohr’s atomic model.
Principle of complementarities
Some experiments indicate that light behaves like a wave; others indicate
that it behaves like a stream of particles. These two theories seem to be
incompatible, but both have been shown to have validity. Physicists finally
came to the conclusion that this duality of light must be accepted as a fact of life.
It is referred to as the wave particle duality. To clarify the situation, the great
Danish physicist Niels Bohr (1885–1962) proposed his famous principle of
complementarity. It states that:
“To understand an experiment, sometimes we find an explanation using
wave theory and sometimes using particle theory. Yet we must be aware
of both the wave and particle aspects of light if we are to have a full
understanding of light.”
We need both to complete our model of nature, but we will never need to use
both at the same time to describe a single part of an occurrence. Therefore
these two aspects of light complement one another. We cannot readily picture
a combination of wave and particle. Instead, we must recognize that the two
aspects of light are different “faces” that light shows to experimenters.
2.1.4. Wave Nature of Matter
In 1924, Louis de Broglie (1892–1987) extended the idea of the wave–particle
duality. He formulated the hypothesis, claiming that all matter, not just light
only, has a wave like nature. He related the wavelength (λ ) and the momentum
(p) by the equation.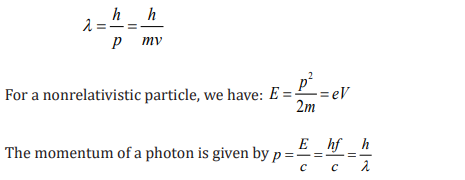
2.1.5. Types of photon Interactions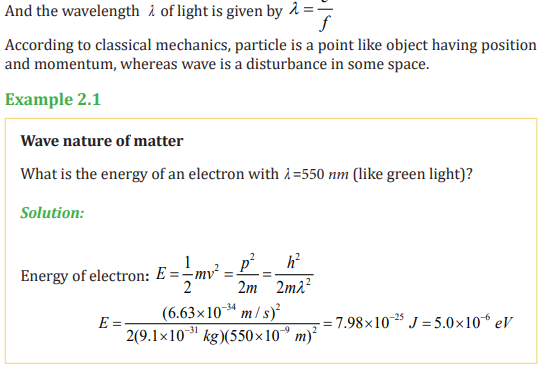
When a photon passes through matter, it interacts with the atoms and electrons.
There are four important types of interactions that a photon can undergo:
1. The photoelectric effect: A photon may knock an electron out of an atom
and in the process the photon disappears. To escape from the surface, an
electron must absorb enough energy from the incident light to overcome
the attraction of positive ions in the material. These attractions constitute
a potential-energy barrier; the light supplies the “kick” that enables the
electron to escape. The photoelectric effect provides convincing evidence
that light is absorbed in the form of photons.
2. The photon may knock an atomic electron to a higher energy state in the
atom if its energy is not sufficient to knock the electron out altogether. In
this process the photon also disappears, and all its energy is given to the
atom. Such an atom is then said to be in an excited state.
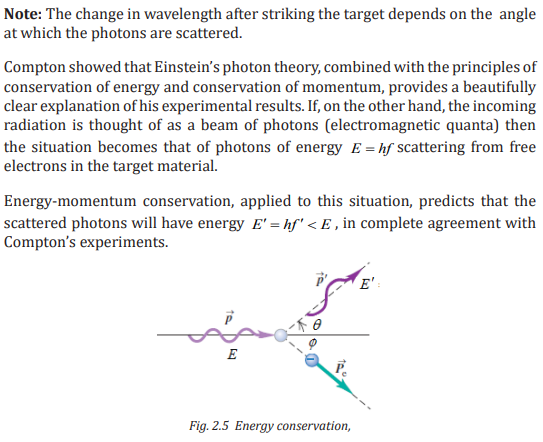
3. Compton Effect: The photon can be scattered from an electron (or a nucleus)
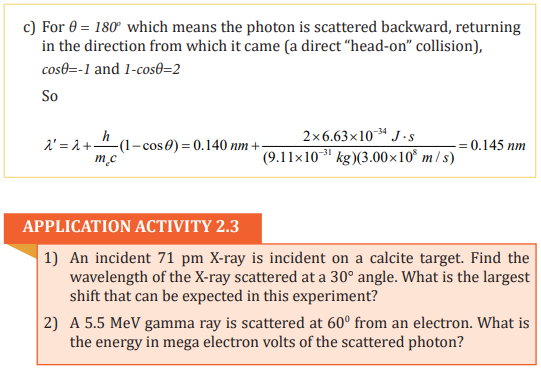
and in the process lose some energy; this is the Compton Effect(Fig. 2.1).
But notice that the photon is not slowed down. It still travels with speed c,but its frequency will be lower because it has lost some energy.
A single photon of wavelength strikes an electron in some material,
knocking it out of its atom. The scattered photon has less energy (some
energy is given to the electron) and hence has a longer wavelength (shown
exaggerated).
4. Pair production: If a gamma-ray photon of sufficiently short wavelength
is fired at a target, it may not scatter. Instead, as depicted in Fig.2.2, it may
disappear completely and be replaced by two new particles: an electron
and a positron (a particle that has the same rest mass as an electron but
has a positive charge rather than the negative charge of the electron).
This process, called pair production, was first observed by the physicists
(Patrick Blackett and Giuseppe Occhialini). The electron and positron haveto be produced in pairs in order to conserve electric charge.
The inverse process, electron–positron pair annihilation, occurs when a
positron and an electron collide.
In pair production, the photon disappears in the process of creating the
electron–positron pair. This is an example of mass being created from pureenergy, and it occurs in accord with Einstein’s equation.


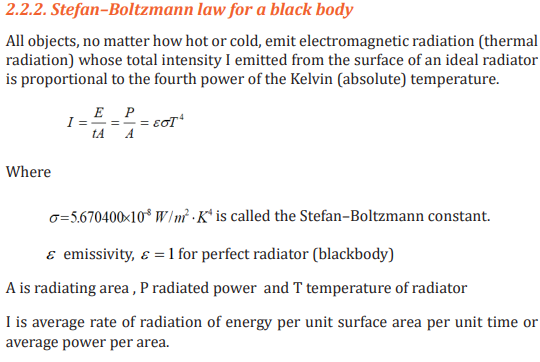
2.2.1. Concept of blackbody
A blackbody is a body that, when cool, would absorb all the radiation falling
on it (and so would appear black under reflection when illuminated by other
sources).
A good approximation to a blackbody is a hollow box with a small aperture in
one wall (Fig. 2.2). Light that enters the aperture will eventually be absorbed
by the walls of the box, so the box is a nearly perfect absorber. Conversely,
when we heat the box, the light that emanates from the aperture is nearly idealblackbody radiation with a continuous spectrum.
Note: When the box is heated, the electromagnetic radiation that emerges from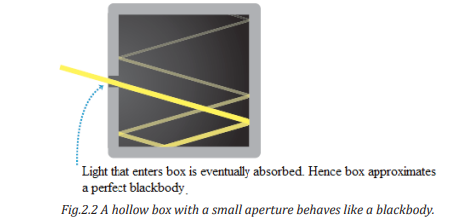
the aperture has a blackbody spectrum.
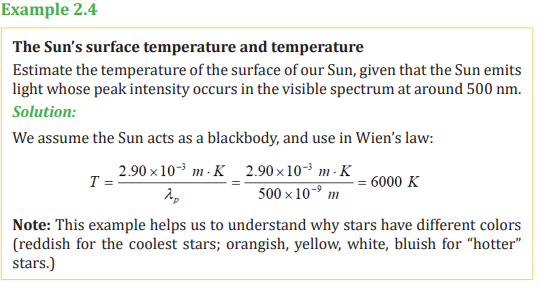
Our sun, which has a surface temperature of about 6000K, appears yellow, while
the cooler star Betelgeuse has a red-orange appearance due to its lower surface
temperature of 2900 K. Our body at 310 K emit electromagnetic radiation in
the infra-red region of the spectrum, and these can be detected with infra-redsensitive devices.

The experimental value of the constant in expression above is 2.90 x 10-3 m.K.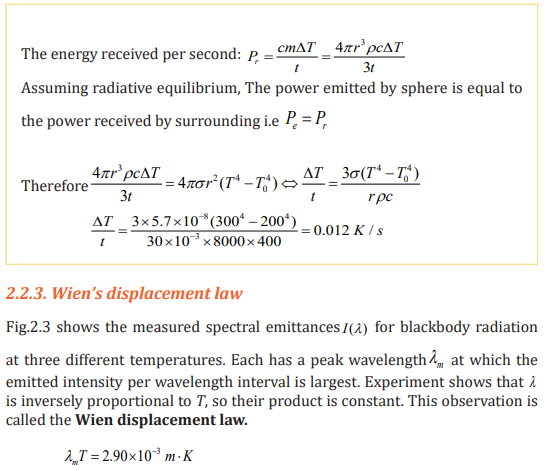
The spectrum of radiation depends on the temperature and the properties of
the object.
At normal temperatures , we are not aware of this electromagnetic radiation
because of its low intensity. At higher temperatures, there is sufficient infrared
radiation that we can feel heat if we are close to the object.
At still higher temperatures (on the order of 1000 K), objects actually glow,
such as a red-hot electric stove burner or the heating element in a toaster. At
temperatures above 2000 K, objects glow with a yellow or whitish color, suchas white-hot iron and the filament of a light bulb.
The spectrum of light emitted by a hot dense object is shown in Fig. 2.3 for an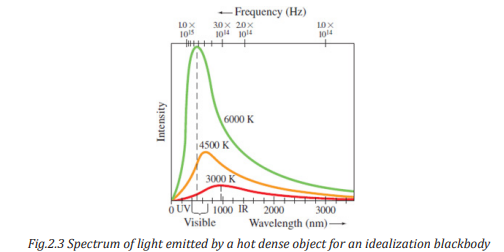
idealized blackbody. The radiation such an idealized blackbody would emit
when hot and luminous, called blackbody radiation (though not necessarily
black in color), and approximates that from many real objects.
The 6000 K curve in Fig. 2.3, corresponding to the temperature of the surface of
the Sun, peaks in the visible part of the spectrum. For lower temperatures, the
total intensity drops considerably and the peak occurs at longer wavelengths
(or lower frequencies).
This is why objects glow with a red color at around 1000 K. Measured spectra
of wavelengths and frequencies emitted by a blackbody at three differenttemperatures.
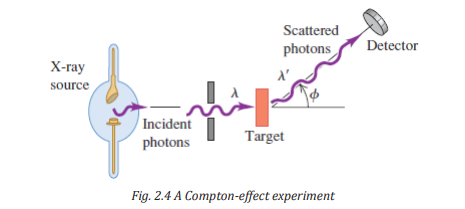
In 1920, Arthur Holly Compton investigated the scattering of monochromatic
x-rays (electromagnetic radiation) from various materials. In his experiment
Compton aimed a beam of x rays at a solid target and measured the wavelength
of the radiation scattered from the target (Fig. 2.4). The incident photon would
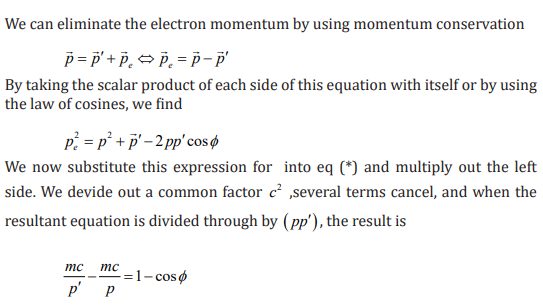
give up part of its energy and momentum to the electron, which recoils as aresult of this impact.
The scattered photon that remains can fly off at a variety of angles θ with
respect to the incident direction, but it has less energy and less momentum
than the incident photon (Fig.2.4).
Therefore, in the photon model, the scattered light has a lower frequency and
longer wavelength than the incident light. This is precisely what the photon
model predicts for light scattered from electrons in the target, a process that isnow called Compton scattering.