General
UNIT 7:Capacity Measurements
Key Unit Competence
A learner should be able to understand capacity, convert between units of
capacity and apply them in solving mathematical problems related to daily life
situations.
Attitudes and values
• Show an ability to properly use a range of materials to measure different
liquids in daily life.
• Being honest and trustworthy when measuring different capacities.
• Being able to show respect for one another when working in groups.
7.1 Understanding Capacity
In our daily conversation, we usually make statements like:• This is a small plane and that is a big plane.
• My cup is small but Dad’s cup is big.

• An orange is smaller than a pineapple.

• A lion is smaller than an elephant.
All the above statements compares the volume or capacity of one object with
another.
Volume is the space occupied by a given object.
Capacity, on the other hand, is the amount of liquid or solid an object can hold.
Activity 7.1
Collect empty containers and compare their capacities by filling water or anyother liquid.
7.2 Units of volume
Consider a container in the form of a cube as shown in the diagram below.
Each side of the container is of length 1 cm.Capacity of the container = length × width × height = 1 cm × 1 cm × 1 cm = 1 cm3.
Activity 7.2
(a) Measure the length (l), width (w), and heightof the matchbox
provided. Record your results in millimetres (mm).
(b) Calculate the volume of one matchbox using the formula;
V = l × w × h.
(c) Pile the matchboxes so as to form a big cuboid of matchboxes.
(d) What is the volume of the big box you have formed?(e) Can you design other different big boxes using the same matchboxes?
Exercise 7.2
1. The figure below shows several small cubes of different colours joinedtogether. Each small cube has a volume of 1 cm3.
(a) How many small cubes are there altogether?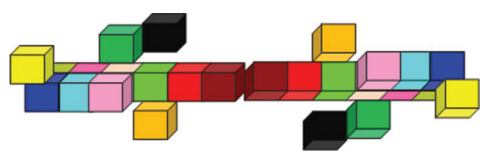
(b) What is the total volume of all the cubes?2. Find the volume of the solids if each cube has a volume of 1 m3
7.3 Estimating capacities of different containers
Activity 7.3
In this class activity, you are going to discuss the statements given with
your classmates. State whether you agree or not. You may experiment (if
possible) in your classroom.
(a) The capacity of a tea spoon is about 5 ml or 5 cm3. The capacity of anordinary glass for drinking water is about 300 ml or 300 cm3 or 0.3 litre.
(b) The capacity of a mug is about 0.5 litre. The capacity of a water jug is
about 2 litres.
(c) The capacity of a small jerrycan is about 5 litres. The capacity of a big
jerrycan is about 20 litres.

(d) A large water tank has a capacity of about 1 000 litres.

Think!!!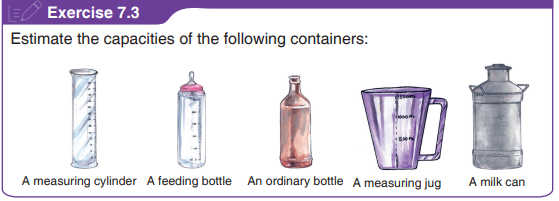
What is full of holes and yet it holds lots of water?
7.4 Conversion of units for capacityConversion table for capacity
We can see that,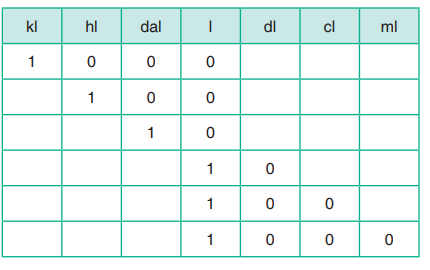
1kilolitre = 1 kl = 1 000 l
One hectolitre = 1 hl = 100 l
One decalitre = 1 dal = 10 l
One litre = 1 l = 10 dl
One decilitre = 1 dl = 0.1 l (a tenth of a litre)
One centilitre = 1 cl = 0.01 l (a hundredth of a litre), e.t.c.
Example 7.1
Change the following units of capacity as instructed below:
(a) 20 l to ml (b) 200 l to hl (c) 20 kl to l
(d) 50 kl to dal (e) 100 dl to ml (f) 35 hl to dal
(g) 7 800 cl to l60 000 ml to l
SolutionWe construct a conversion table to enable us convert these units.
(a) 20 l = 20 000 ml (b) 200 l = 2 hl (c) 20 kl = 20 000 l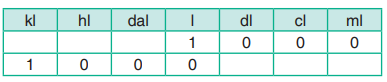
(d) 50 kl = 5 000 dal (e) 100 dl = 10 000 ml (f) 35 hl = 350 dal
(g) 7 800 cl = 78 l60 000 ml = 60 l
Activity 7.4
A cow produced milk as follows;
Monday - 2 litres
Tuesday - 5 litres
Wednesday - 3 litresHow much milk did it produce in three days? Write answer in ml.
7.5 Addition and Subtraction of Capacity
Activity 7.5
In groups, do the following:
• Measure the capacity of water in a jug.
• Change this into litres.
• Now, measure the capacity of a bucket by pouring water using 1 litre
bottles.
• Compare the capacities. Which has more water? Obtain the total volume
of water in litre.
Example 7.2
(a) Add: 900 decalitres + 400 litres(b) Subtract: 24 litres – 2 400 centilitres
24 l – 2 400 cl = 2 400 cl – 2 400 cl = 0.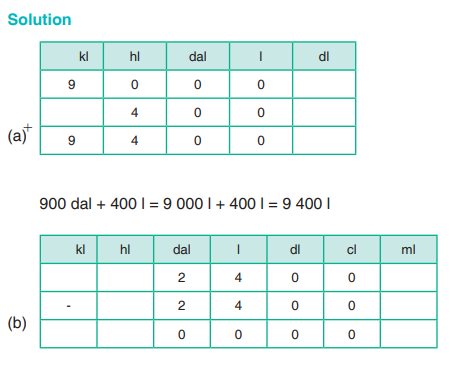
Example 7.3
(a) 7.50 l + 3.50 l(b) 6.30 l – 2.30 l
7.6 Application of Capacity
Example 7.4
1. A bottle of mineral water has a capacity of 300 ml. How many bottles of
mineral water do I need to make 3 litres?
2. Mutoni sells 11 cans of milk to a milk depot every week. If each can
of milk has a capacity of 20 litres, how much milk does she sell per
month?
Solution
1. 3 l = 1 000 × 3 = 3 000 ml. Since 3 l = 3 000 ml, therefore, the number
of mineral water bottles is given by:
3000 ml ÷ 300 ml = 10
2. Number of cans sold per week = 11
Number of cans sold per month = 11 × 4 = 44 cans (1 month = 4 weeks)Quantity of milk sold in 1 month = 44 × 20 litres = 880 litres.
Activity 7.6Study the following figure and answer the given questions:
1. Find how much water should be added so as to fill up the tank fully.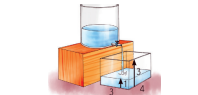
2. How much water was there earlier in the tank. (Dimensions shown infigure are in centimetres)
Assessment Exercise
1. Add: (a) 3 l 500 ml + 5 l 700 ml (b) 3.25 l + 6.75 l
2. Subtract: (a) 5 l 400 ml – 3 l 250 ml (b) 10.5 l – 2.75 l
3. A restaurant sells 200 l of milk every day. How much milk is sold in
3 months? (assume 1 month = 30 days).
4. A school water tank contains 12 000 l of water. Pupils in the school
used 40 hectolitres of water. What amount of water is remaining?
5. The capacity of a milk jug is 750 ml. By how much is this less than a
litre?
6. The cost of milk is 150 Frw per mug (cup). Two such cups make up one
litre of milk. How much will a person spend on 4 litres of milk?
7. On average, each pupil takes 1.5 litres of milk in our school. How much
milk is taken by 100 pupils?8. A Friesian cow in a certain farm produces
15 litres of milk in the morning and 10 litres
of milk in the evening. How much milk does
it produce in a week?
9. The price of petrol is 900 Frw per litre. A school bus requires 100 litresof petrol for a school trip. How much is spent on petrol?
Internet ResourceFor more online support visit www.math-play.com/capacity
